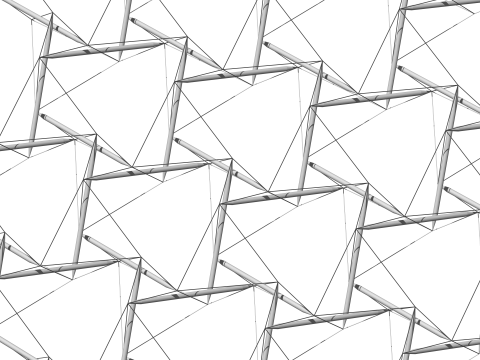
Figure A.1: Snelson's Planar Truss
A Practical Guide to Tensegrity Design
Table of Contents
Appendix A
Other Double-Layer Technologies
Two other approaches to planar tensegrity truss design similar to the approach outlined in Chapter 5 have been independently developed by Kenneth Snelson1 and a group of authors consisting of David Georges Emmerich2, Ariel Hanaor3 and René Motro.4 For the most part, the tendon network for the outer and inner layers of these structures is identical with the double-layer truss described in Chapter 5. However, the way members are connected between the layers is different, and neither of these alternative approaches have been elaborated for use in a spherical context by their authors.
A planar truss from Kenneth Snelson's work is the most similar to the trusses exhibited in Chapter 5. It only differs in the number and connection of the interlayer tendons. Figure A.1 exhibits a rendering of Snelson's truss as it would appear in an infinite planar context. For each strut, there is a corresponding interlayer tendon which makes a fairly direct connection between the two layers and skirts the strut closely. The close approach of the strut and the interlayer tendon makes the use of the truss in a spherical context difficult due to interference problems. A more usable approach for spherical contexts might be obtainable by threading the interlayer tendon through the middle of the corresponding strut.
 Figure A.1: Snelson's Planar Truss |
The starting point of Emmerich et al.'s system is a planar assembly of t-prisms.5 Figure A.2 shows how such a planar assembly would appear.6 As is evident in comparing Figures 2.1 and 5.1, the topological difference between a t-prism and a t-tripod is not great: the t-prism is topologically equivalent to a t-tripod with a tendon triangle connecting the struts at the t-tripod's base.7 While the manner of Emmerich et al.'s assembly retains triangulation in the outer and inner tendon layers, the interlayer triangulation exhibited by individual prisms is broken up by the arrangement. In this situation a shallow dome can be induced by introducing curvature in the planar assembly of prisms by transforming the prisms into truncated pyramids.
 Figure A.2: Planar Assembly of T-Prisms |
Figure A.3 shows how a truss based on t-tripods looks in a planar context. Its appearance is most similar to Snelson's truss, the only thing differentiating them being that there are two interlayer tendons corresponding to each strut in the truss based on t-tripods whereas Snelson's truss just has one. In the t-tripod truss, the way the tendons are connected in relation to the strut avoids interference problems with that strut; however, interference with other struts is always a possibility which may have to be designed around. As seen in Chapter 5, the outer layer has been completed by interconnecting the t-tripod apexes with tendon triangles to yield an outer layer which is also identical with that obtained in Emmerich et al.'s arrangement.
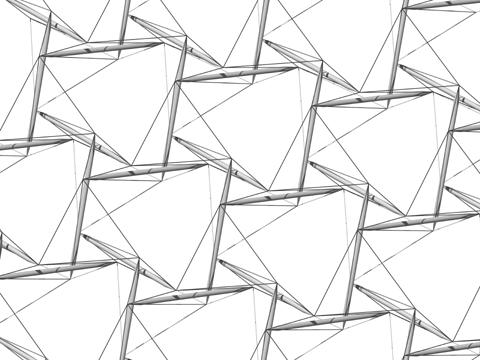 Figure A.3: Planar Assembly of T-Tripods |
Each inner tendon triangle where the struts of three t-tripods converge is viewed as the apex of an inward-pointing t-tripod. Adding the corresponding interlayer tendons to complete these t-tripods (the secondary interlayer tendons of Chapter 5) provides more interlayer triangulation, and thus more reinforcement of the structure. In this configuration, each strut is secured by 12 tendons. It is worth noting that this is precisely the minimum number of tendons Fuller has experimentally found to be necessary to rigidly fix one system in its relationship to a surrounding system.8
Hanaor's articles also present computations and models for several structures. Computations for both member lengths and forces are presented. All computations are based on a methodology presented in Argyris72. An important qualification to these results is to notice that only one layer of the truss is secured to a rigid support whereas, for best performance, both layers should be. This is difficult unless the truss has been elaborated into a hemisphere of some kind. The structural behavior of Snelson's approach has not been explored with civil engineering tools. The relative performance of these two methodologies and the one presented in this work is unknown.
1 See photos in Lalvani96, p. 48. A realization of his truss described here was embodied as his Triangle Planar Piece Model of 1961. It also appears in Figures 7 and 8 of some 1962 drawings which are part of an abandoned patent application.
2 Emmerich88, "reseaux antiprismatiques", p. 281.
5 This is a typical application of the technique. It has various ways it can be applied, and is not limited to t-prisms.
7 Hanaor might call the t-tripod a truncated pyramid with its larger end triangle removed. See Hanaor92, Fig. 3(f).