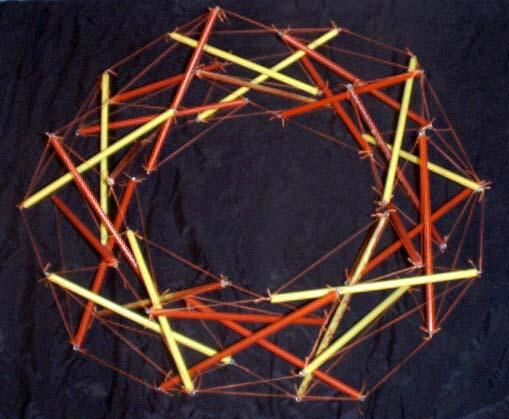
I finished this model of a ten-stage tensegrity torus on December 24, 2003. I had put together a basic design at the beginning of the month. During my original investigations, I found the ten-stage torus to be the torus with the smallest number of stages that didn't have interference among the members. My first version of an eight-stage torus had interference problems, but later (February, 2004) I found a few parameter tweaks yielded a model which could be assembled. Having resolved those interference problems, I also developed assemblable models of a six-stage version and a four-stage version.

Designing and building a torus would have been a logical prelude to building the arch, but I derived that from a tower design. I think my leap-frogging was for the best since otherwise I don't think I would have come up with the attractive streamlining that resulted when I derived the arch from a tower. Now that I've done the torus though, I think it would be interesting to design some torus-derived arches. My assembly experience indicates an interesting seven-stage arch could be derived from the ten-stage design, but I imagine I'll try to develop a small arch from one of the lesser-stage tori.

I assembled the torus in stages. The first stage was the most difficult. Finally, following a suggestion by Doug Milliken, I used a jig consisting of a one-gallon jug of cylindrical shape to help me assemble the first stage. Tieing the first hexagon of "tS/TS" tendons with three struts pointing one way and three struts going the other way I did without the jig. The jig was introduced to tie on another hexagon of "tS/TS" tendons at the other end of the stage and introduce another three struts. I used two rubber bands to secure the first six struts to the jug. Then tieing in the next three struts with the second "tS/TS" hexagon was fairly easy. The next step for the first stage was tieing in the "guy" tendons. To tie in the third "guy" tendon I needed to slide the stage off the jig. From then on the jig wasn't necessary. The last step for the first stage was to tie the "tT" tendons. I then had a somewhat-limp prism with three additional struts corresponding to adjacent stages attached at either end.

Assembling each stage consisted of first introducing the "guy" tendons. Then the hexagon of "tS/TS" tendons was introduced which also introduced three struts from an adjacent stage. Finally the "tT" tendons were tied in connecting the struts of the two adjacent stages.

Once I started assembly, I realized the torus had a reflective symmetry which I rarely observe in the tensegrities which I construct. Such a symmetry might be more frequent in tensegrities which have strut-strut connections. The only other tensegrity without strut-strut connections where I've observed this type of symmetry is Buckminster Fuller's tensegrity icosahedron (see figure 270 in The Dymaxion World of Buckminster Fuller by R. Buckminster Fuller and Robert Marks).

In this torus, adjacent prism stages have opposite twists. This is true of most of the linear tensegrities based on prisms I've seen with the exception of Maxim Schrogin's arch. For one set of five stages, I used struts colored Chinese red. For the other set of five stages I used prisms consisting of two yellow struts and one red strut spattered with yellow. The latter red/yellow strut I positioned toward the center of the torus.

I tried some torus designs with prisms twisting only one way, but couldn't come up with anything satisfactory though I didn't work too hard at it. I got an interesting result for one problem which yielded two solutions, one exhibiting five-fold symmetry (but not reflective symmetry) and the other a peculiar-looking structure which was asymmetric. Both I imagine are valid tensegrities, but this is the first time I had one tensegrity mathematical programming problem yield two solutions.

In later research, I found David Emmerich presented a tensegrity torus with a topology very similar to what I use here in his 1988 book Structures Tendues et Autotendantes (Prestressed Tensile Structures -- see his "tore interpenetre hexagonal moeubiussien trapezoide" on pp. 264-265), though his torus seems like a preliminary design such as I would use to generate initial coordinates for my tensegrity software. In addition, his prisms don't alternate in sense as one goes around the torus like the ones above do. I was surprised I couldn't find an example with alternating-sense prisms. Just an inadvertent ommission I imagine. He has so many interesting variations, and I hope to explore them in more detail at some point.

All these designs are available as VRML models.