A tensegrity prism can be skewed along its central axis and the resulting structure will still be a tensegrity. In addition, for a prism with equilateral ends, the pattern of base forces is not affected by the skew transformation. These results are described in a paper I wrote on skew prisms. The application of these results to a variation on John Moelman's tensegrity vector equilibrium allowed the simple computation of the structure above. Forces in all members are proportional to their lengths. Aside from the ends, there are three flat sides which it can rest on.
For me, this structure has a chapel-like quality and brings to mind Albrecht Dürer's drawing Praying Hands. This structure is available as "Skew Tensegrity" in all the tensegrity viewers. I completed this design on January 27, 2006, and put together a datasheet on August 24, 2007, a photo of which is above. I assembled a model on August 27, 2007. Assembling the model emphasized the simplicity of the structure (considering that it's a skew design): while there are three different strut sizes, there are only two different tendon lengths, and all member stresses are proportional to the lengths.
Below is an arch designed using the same skewing procedure. This structure is available as "Skew Prism Arch" in all the tensegrity viewers. I completed this design on February 8, 2006, and put together a datasheet on August 27, 2007. I completed a model using this datasheet on September 10, 2007, a photo of which is below:
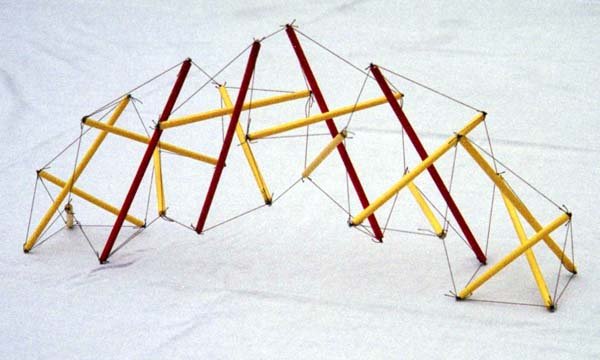
Dick Fischbeck, in a comment on the Geodesic list November 10, 2007, noted the central hexagon might act like a diaphragm, and wondered if the hexagon was regular. In my response of November 11, I noted there was a whole string of regular hexagons in the structure and emphasized the design simplicities of this particular tensegrity technology:
Diaphragm is a good way to describe it. All the hexagons are planar regular hexagons which is what makes it easy to do the computations for the structure. The planes for all the hexagons and triangles are parallel. The gaps between the planes are all equal, though they don't need to be and any way of spacing the gaps would work. The same goes for the positions of the triangles and hexagons in their respective planes. They could be shifted to any location in their respective planes and a valid tensegrity would still result. The orientation, relative twist, is all that needs to be preserved. The triangles and hexagons can also be scaled to arbitrary relative sizes, just keep them regular.
I imagine there are more flexibilities that apply, but I haven't worked through the intricacies of what happens when irregular polygons are used.
Dick responded that day with a mark up of my photo which more clearly emphasized the hexagons.
The skew transformation is actually a special case of the result in Milenko Masic, Robert E. Skelton and Philip E. Gill's article "Algebraic tensegrity form-finding", International Journal of Solids and Structures, Vol. 42, Nos. 16-17 (Aug 2005), pp. 4833-4858. They show that tensegrity equilibrium is preserved by affine transformations (a linear transformation plus a translation). This covers skew transformations as well as many other interesting ones. I think the ability to do the step-wise skew transforms on a structure as shown here is a slightly stronger property. For example, I was unsuccessful in doing a step-wise skew transform for Snelson's Tensegrity Tower, but I haven't given up.