VRML Model

I completed assembling this figure on June 1, 1997. It is a variation on the 2v Double-Layer Tensegrity Octahedron. Here the tendons which connect the two layers connect the outer points directly with the corresponding inner points. (The outer layer is like a blown-up version of the inner layer except that it has two sizes of triangles rather than eight triangles of equal size.) The result is shorter interlayer tendons. The twelve struts are thus no longer organized as four tensegrity tripods. This made the structure more difficult to assemble.
This structure felt about as stiff to me as the earlier structure. I thought with less triangulation it might be less rigid, but maybe the more-direct connection between the layers makes up for that.
This piece was shown at Black Mountain College Museum and Art Center as part of the exhibition "Ideas + Inventions: Buckminster Fuller and Black Mountain College" (July 15 - November 26, 2005):

It was nice to see it in such distinguished company. Rupert Ravens of nices inc., the sponsor of the exhibition, purchased the structure in June, 2006.
The difference between this 2ν variation and the original is analagous to the difference between a truss Snelson developed, and the one I use. Snelson's truss uses a single interlayer tendon per strut which makes a direct connection between the two layers. A diagram of Snelson's truss (more views can be found at Snelson's Planar Pieces -- see Figures 7 and 8 in particular):
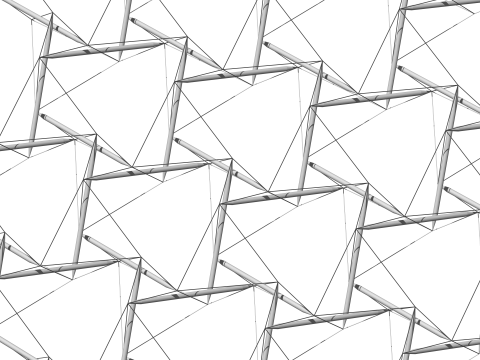
It is remarkable to me that the interlayer tendon actually clears the strut though it skirts it very closely. In general, this truss is difficult to use in higher-frequency spheres (though as seen above it works fine at 2ν) due to the intractable interference between the strut and its corresponding tendon. (This might be obviated by actually threading the tendon through the strut.) It is curious that the interference problems disappear at the two extreme manifestations of the truss, the 2ν sphere in the photos above and the planar truss directly above.
A diagram of the truss I used in the original 2ν sphere (except, as noted, the planar truss has both of the interlayer tendons corresponding to each strut):
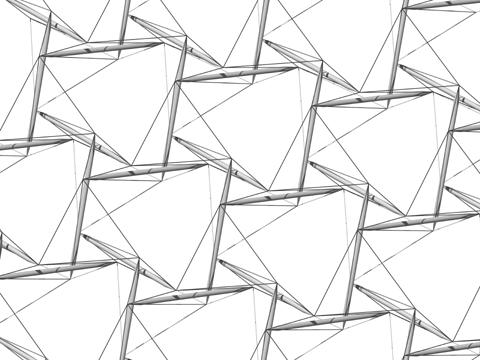
Note that both this truss and Snelson's truss have rotational symmetry axes in the plane of the truss (two-fold) as well as perpendicular to the plane of the truss (three-fold).