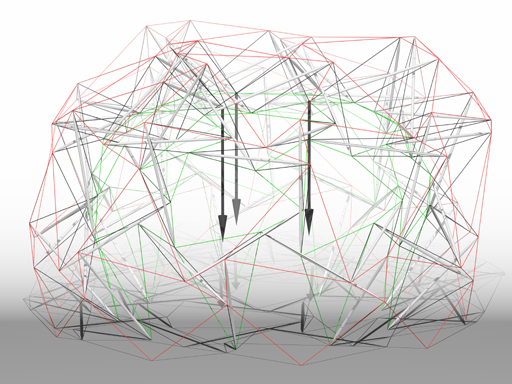
Figure 7.1: 6ν T-Octahedron Dome: Positions and Effect of Exogenous Loads
A Practical Guide to Tensegrity Design
Table of Contents
7.2 Endogenous Member Forces
7.3 Exogenous Member Forces
7.3.1 Exogenous Force Analysis: Method
The analysis of the response of a tensegrity structure to exogenous forces is achieved with a change of conceptual framework. A structure is now viewed as a flexibly-jointed set of elastic and fixed-length members: the tendons being the elastic components, and the struts being the fixed-length components. Initially it is assumed that the hub is a single point. In Section 7.3.5, this assumption is relaxed.
The solution of the tensegrity programming problem and the subsequent endogenous force analysis provide a valid initial unloaded configuration for these members, a valid configuration being one in which the net force at each hub is zero. The unloaded forces at each hub are tendons pulling in various directions, a single pushing strut and pulling or pushing reactions due to any point constraints. The reaction due to a point constraint is in the direction of the determining vector of the constraint.
An exogenous load is introduced at selected hubs by adding an independent force vector to the forces present at a hub. In the initial configuration, the net force at these hubs is no longer zero, and a new configuration of the structure must now be found in which the net force at each hub is again restored to zero. A new configuration is derived by solving a system of equations rather than by solving an extremal problem as before. There is one equation each for the , and component of the net force at each hub. This value must be equated to zero. Then there is one equation each for the length of each fixed-length member. This length must not change in the new configuration.
These equations are non-linear in their variables. The variables are the coordinate values, the forces in the fixed-length members and the scalings for the reactions due to the point constraints. (The force in an elastic member is determined by the coordinates of its end points and the elasticity equations which govern the member; so, it is not an equation variable.) The system is solved using the standard Newton method. The exogenous load forces may need to be introduced in an incremental way in order for the Newton method to converge.
7.3.2 Exogenous Force Analysis: Mathematical Framework
Two sets of equations must be satisfied for any tensegrity configuration. The first set of equations constrains the forces at the hubs to balance to zero. The net force at a hub is the sum of the forces in the members that meet at that hub, plus the sum of the reactions due to point constraints which impact the hub plus any exogenous force at the hub. The force due to a member will have a magnitude corresponding to the force in the member and a direction corresponding to the orientation of the member. The reaction force due to a point constraint is the determining vector of the constraint multiplied by a scaling factor which is a variable of the analysis. For a strut, the force is into the hub along the length of the strut; for a tendon, the force is out of the hub along the length of the tendon. So, the first set of equations is:
where:
|
=
number of hubs = number of members meeting at hub = number of point constraints impacting hub = force at hub due to member (vector) = index of the member meeting at hub = force at hub due to point constraint (vector) = index of the point constraint impacting hub = exogenous force at hub (fixed vector) |
The formula for is:
where:
|
= signed magnitude of force at hub
due to member
(vector) = point corresponding to hub = end point of member away from hub |
If member is fixed-length (i.e. a strut), then is a negative variable whose value is adjusted to obtain a solution. If member is elastic (i.e. a tendon), then
when and otherwise, where:
|
= proportional elasticity coefficient for member
10 = reference length for member |
The formula for is:
where:
|
= scaling value for reaction force at hub
due to point constraint
= determining vector for point constraint |
is a variable whose value is adjusted to obtain a solution.
The second set of equations is just the point constraints.
The third and last set of equations constrains the lengths of the struts to remain constant:
where:
|
= number of struts =
= index of the strut = difference vector for the end points of member (order of subtraction not important) |
Thus, there are equations which must be solved for the coordinate values of the hub points, the scaling values for the reaction forces corresponding to the point constraints and the magnitudes of the forces in the struts.
7.3.3 Exogenous Force Analysis: Initialization
An initial solution for these equations in the absence of exogenous loads can be obtained from coordinate values and endogenous forces computed using the methods described in Sections 7.2.3 and 7.2.4. The hubs are positioned according to the coordinate values. For the struts and point constraints, the force values obtained from the endogenous analysis are used to initialize and . For each tendon, a value for is chosen in accordance with the material being used for the tendon. is then chosen to be sufficiently smaller than the minimizing length of the tendon so that the value of is equal to the force for the tendon obtained from the endogenous analysis. Once this initial solution is obtained, values for are incrementally introduced at the appropriate hubs, and the system is solved using Newton's method at each increment. If the Newton iterations diverge at any point, a smaller increment can be chosen until the iterations converge.
7.3.4 Exogenous Force Analysis: A Sample Calculation
This methodology can be used to analyze the response of the 6ν t-octahedron dome (designed in Section 6.2) to an exogenous load. To reduce the computation required, the load will be applied symmetrically to the structure. The hub corresponding to and the two hubs symmetric to it will be loaded with a relative value of . This is a force vector pointed toward the base of the structure. It is diagrammed in Figure 7.1.
The first step is to choose suitable values for the and parameters. is chosen to be the same for all tendons, and so that, when the average endogenous tendon force is applied to a tendon, it elongates by 2%. The average value for the endogenous force over all the tendons is 3.1294. This is computed from Tables 6.28 to 6.31. Therefore, (the common value of all the parameters) is chosen to be or 156.47.
Note that, for this sample calculation, all forces are posed in relative terms. To get real values, everything would need to be scaled. For example, if the tendons for the 6ν t-octahedron dome were composed of a material such that a force of 20 pounds (89 Newtons) is required to elongate a tendon by 2%, all force values would be scaled by . This would make the magnitude of the exogenous load = 33.21 pounds (147.7 Newtons). The scale factor would also be applied to Tables 7.6 to 7.10 to get values in pounds.
Given the value for , the values for the parameters are chosen so the initial tendon forces match the computed endogenous forces. Tables 7.2 to 7.5 summarize the values used. As always, excluded members are marked with ‡.
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
Table 7.2: 6ν T-Octahedron Dome: Primary and Secondary Interlayer Tendon Reference Lengths |
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
Table 7.3: 6ν T-Octahedron Dome: Inner and Outer Convergence Tendon Reference Lengths |
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
Table 7.4: 6ν T-Octahedron Dome: Outer and Inner Binding Tendon Reference Lengths |
| ||||||||||||||
|
Table 7.5: 6ν T-Octahedron Dome: Guy Reference Lengths |
The system is solved using the numerical version of the Newton method with a value of 0.001 for the double-sided numerical differentiation differential. Iterations are done until equations are solved within . This requires 21 iterations.
Figure 7.1 shows the positions and effect of the exogenous loads on the dome. Table 7.6 summarizes the resultant forces in the struts. Tables 7.7 to 7.10 summarize the resultant lengths and forces for the tendons. Tables 7.11 and 7.12 summarize the resultant coordinate values. Tables 7.13 and 7.14 summarize the resultant force vectors at the fixed base hubs before and after the load is applied. For the guy attachment points (, and ) the sum of the component values of the force vectors at each point is positive. This means a force upward from the base is being exerted at those points. This is as expected since only tendons from above the base are attached at those points. descends by 0.66659 model units from 5.60914 units above the base of the structure to 4.94255 units as a result of the exogenous load. Notice also that in Table 7.9 a number of the binding tendons have gone slack. It might also be worthwhile to check clearances to see if any of them have been affected adversely by the load. The assumptions of these calculations would be violated if the exogenous load drove one member into or through another.

Figure 7.1: 6ν T-Octahedron Dome: Positions and Effect of Exogenous Loads |
| ||||||||||||||||||||||||||||||||||||||||||||||||||||
|
Table 7.6: 6ν T-Octahedron Dome: Strut Loaded Forces |
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
Table 7.7: 6ν T-Octahedron Dome: Primary and Secondary Interlayer Tendon Loaded Lengths and Forces |
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
Table 7.8: 6ν T-Octahedron Dome: Inner and Outer Convergence Tendon Loaded Lengths and Forces |
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
Table 7.9: 6ν T-Octahedron Dome: Outer and Inner Binding Tendon Loaded Lengths and Forces |
| |||||||||||||||||||||
|
Table 7.10: 6ν T-Octahedron Dome: Guy Loaded Lengths and Forces |
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
Table 7.11: 6ν T-Octahedron Dome: Loaded Inner Coordinate Values |
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
Table 7.12: 6ν T-Octahedron Dome: Loaded Outer Coordinate Values |
| ||||||||||||||||||||||||||||||||||
|
Table 7.13: 6ν T-Octahedron Dome: Base Point Unloaded Force Vectors |
| ||||||||||||||||||||||||||||||||||
|
Table 7.14: 6ν T-Octahedron Dome: Base Point Loaded Force Vectors |
7.3.5 Exogenous Force Analysis: Complex Hubs
The previously-outlined technique for exogenous force analysis works when vector constraints are not being used and the simple assumption that the hubs of the tensegrity are single points is being made. When hubs are complex and thus vector constraints are introduced, torque considerations must also be introduced. For this latter situation, the model which follows is proposed.
In the new model, corresponding to every strut is a strut envelope. The strut envelope is a single rigid body to which tendons are attached and which also may be impacted by point constraints and exogenous forces. Tendons, point constraints and exogenous forces are all assumed to impact the strut envelope at single points distributed over the envelope. These points are referred to as attachment points. The shape of the strut envelope is determined by the strut equations and the vector constraints. The strut equations are incorporated as constraints which maintain the struts at fixed lengths. There must be a sufficient number of strut and vector constraints so that the strut envelope is rigidly determined.
In contrast to the previous model where tendons were only attached at one of two points on the strut, now each tendon can have a unique attachment point on the strut envelope. It is possible that tendons share attachment points, but they don't need to. All the attachment points, including those for point constraints and exogenous forces, are assumed to cluster at two hubs. Each hub has a corresponding reference point which is referred to as an end point of the strut though the physical strut may extend considerably past it and perhaps not even through it. There must be more than one attachment point at each hub. Joints are still assumed to be flexible, so torque must only be considered for the strut envelope and can be neglected as far as the tendons are concerned.
Forces must still sum to zero in this new model, but only for the strut envelope as a whole rather than for each of the two hubs. In addition, the sum of the torque moments on the strut envelope exerted by all the forces must be zero.11 A force and a torque moment vector can be derived for each strut envelope from the results of the exogenous analysis. These vectors will most likely approximate the axis of the strut, but in many cases will not coincide with it.
The equations representing the requirement that forces sum to zero are now:
where:
|
=
number of struts =
= number of tendons attached to strut envelope = number of point constraints impacting strut envelope = number of exogenous forces impacting strut envelope = force at strut envelope due to tendon (vector) = index of the tendon attached to strut envelope = force at strut envelope due to point constraint (vector) = index of the point constraint impacting strut envelope = th exogenous force impacting strut envelope (fixed vector) |
The formula for is:
where:
|
= signed magnitude of force at strut envelope
due to tendon
(vector) = point where tendon is attached to strut envelope = far attachment point of tendon |
The value of for tendons is derived as before. The value for struts is not relevant since they are not included here. The formula for remains the same and the values are again one portion of the values which are adjusted to solve the system of equations.
The equations representing the requirement that torques sum to zero are:
where:
|
= point on strut envelope
constrained by point constraint
= point on strut envelope where the exogenous force is applied = = center point of strut envelope |
The point constraints must also be met and are now joined by the vector constraints. The the set of equations constraining the lengths of the struts to remain constant are retained as well. Thus, there are equations which must be solved for the coordinate values of and the scaling values, , for the reaction forces corresponding to the point constraints. A necessary condition for this to be possible is that . Since , this necessary condition can be expressed as .
Actually, it is more pertinent to examine this last condition for each strut envelope. For an individual strut envelope, the condition can be expressed as where is the number of vector constraints pertaining to strut and is the number of vectors used to model its hubs. If there are sufficient vector constraints to rigidly determine the strut envelope, this condition should obtain; otherwise, additional vector constraints will need to be added. It is possible that some vector constraints will only be used for the analysis of exogenous loads and will be ignored during the solution of the mathematical programming problem corresponding to the structure.
Once the equations have been solved, characteristic member forces can be computed for the strut envelopes. For each strut envelope, the attachments are separated into two groups corresponding to the two hubs. This is done using the center point of the strut envelope. First, the dot product of the center point is taken with the vector corresponding to the difference of the end points of the strut. Then, the dot product of this difference vector with each attachment point is taken. If the dot product for an attachment point is less than the dot product for the center point, then the attachments corresponding to the point go in one group; if not, they go in the other group. The characteristic member force for the strut envelope is found by summing the forces for one of the two groups of attachments. Since the sum of the forces for all the attachments to the strut envelope is zero, the sum for one group will be the additive inverse of the other group.
In addition, a torque moment can be computed for each strut to estimate the twisting force it is subjected to. This computation uses the standard procedures for computing the moment of forces about a specified axis.12 Using the same procedure as that described for the characteristic member-force computation for the strut envelopes, the attachments are separated into groups corresponding to the hubs. The signed magnitude of the moments corresponding to each hub are then computed using the following triple scalar products:
where:
|
(vector) = point where strut is attached to hub = far attachment point of strut attached to hub = number of tendons attached to hub = number of point constraints impacting hub = number of exogenous loads impacting hub = force at hub due to tendon (vector) = index of the tendon attached to hub = point where tendon is attached to hub = force at hub due to point constraint (vector) = index of the point constraint impacting hub = point on hub constrained by point constraint = exogenous force impacting hub (fixed vector) = point on hub where the th exogenous load is applied |
Since for the strut as a whole the sum of the moments is zero, the moments for each of the two hubs of a strut will be equal.
7.3.6 Exogenous Force Analysis: Another Sample Calculation
For an example of exogenous force analysis with non-point hubs, it is useful to turn back to the tensegrity prism of Section 2.2. In the course of the example, meta-constraints are also illustrated. Meta-constraints are a design tool which allow a tensegrity to meet certain geometric specifications which would be illegitimate if they appeared in the mathematical programming problem. In this case, the meta-constraint will be that the struts of the prism are at 90° to each other. This allows the prism to be used as a joint in a cubic lattice. If this constraint were imposed in the mathematical programming problem it would be illegitimate and lead to a structure with loose tendons most of the time; however, the desired geometry can be achieved if the constraint is applied at a higher level.
Since the exogenous load is applied asymmetrically, symmetry transformations are not used in the model. For the struts, 14-inch (356 mm) lengths of one-inch (25 mm) square wood stock are used. Holes for attaching the tendons to the strut are drilled at one inch (25 mm) from either end of the strut, so these attachment points are 12 inches (305 mm) apart. So, the model is:
where:
|
;
;
; ; ; ; ; ; ; ; ; ; ; ; ; ; |
= 0.5 inches (13 mm) since it represents half the length of the holes drilled through the wooden struts. The vectors used to construct the offsets from the strut end points to where the tendons are connected to the strut are restricted to be orthogonal to their corresponding struts since the holes are drilled orthogonal to the strut. Note that for each strut the same vector is used to construct all four offsets at the two hubs. This is appropriate since the holes drilled through the struts are aligned with each other. In another situation, a different independently-adjustable vector might be used to construct each offset.
= 12 inches (305 mm) since it represents the distance between the two holes drilled through each wooden strut. will start out at = 5.14 inches (130 mm) and will be adjusted between successive solutions to the mathematical programming problem to obtain a structure with orthogonal struts. It is scaled up from the value of 3 used in Section 2.2.2 to account for the fact that the strut length is now 12 inches (305 mm) rather than 7 inches (178 mm).
Though the model used is more in the vein of the Cartesian version of the tensegrity prism presented in Section 2.2.3. initial data for the mathematical programming problem can be obtained from the results of Section 2.2.2. First the base triangle is placed in a way to satisfy the point constraints. The other end triangle is obtained by rotating the first by 150° about its center and raising it by the appropriate height. The height, which represents the value for z in this model, is found by solving the formula for of Section 2.2.2 for h and using the fact that :
Table 7.15 summarizes the initial values. The initial data fit the constraints closely enough that no penalty iterations are necessary to reach a point so that the equation system can be solved for the dependent in terms of the independent coordinates. The initial iterations are rough in that the step size and the partitioning have to constantly be adjusted to make progress. Initially eight steepest-descent iterations are done and the coordinates are repartitioned at each step. Then eight Fletcher-Reeves iterations are done with no repartitioning necessary. Finally a Newton iteration is done to enhance the accuracy of the solution.
| ||||||||||||||||||||||||||||||||||||||||||||
|
Table 7.15: T-Prism: Initial Cartesian Coordinates | ||||||||||||||||||||||||||||||||||||||||||||
To track the angle between adjacent struts, a dot product is taken of their corresponding vectors. A value of zero indicates that the desired orthogonality is reached. For this first solution, the value is 80.7266. Increasing the value of by 0.01 decreases the dot product to 80.5217. Using the usual Newton technique, this result is used to extrapolate the increase to which added to the original value of yields 9.08266.
This new value for is a large enough change that the equation system can no longer be solved for the dependent coordinates in terms of the independent ones, so ten Fletcher-Reeves iterations are done using the penalty method. This results in a solvable system, but again one in which the initial iterations are rough. A solution is reached which yields a value of -20.2664 for the dot product of the struts. The Newton meta-iterations are continued until a dot product close to zero is reached. Table 7.16 summarizes the sequence of values. None of the changes after the first large one is large enough that the equation system becomes unsolvable, so the exact technique can be used throughout rather than resorting to the penalty method. The final value for is 8.38288, and that for , and is 6.65618.
| ||||||||||||||||||||
|
Table 7.16: T-Prism: Meta-Iteration Values |
The meta-solution values for the control variables are summarized in Table 7.17, and the resulting structure is shown in Figure 7.2. The orthogonal configuration gives the struts their maximum clearance per unit length with respect to each other. Each strut has an edge which is exactly flush with the supporting surface, though this is not peculiar to the orthogonal configuration.
| ||||||||||||||||||||||||||||||||||||||||||||
|
Table 7.17: Orthogonal T-Prism: Cartesian Coordinates | ||||||||||||||||||||||||||||||||||||||||||||

Figure 7.2: Orthogonal Tensegrity Prism |
Table 7.18 summarizes the prestress forces. The prestress forces are scaled so the average tendon force is 20 pounds (89 Newtons). This results in a torque on a strut of 0.75 foot-pounds (1.01 Newton-meters).
| ||||||||||||||
|
Table 7.18: Orthogonal T-Prism: Prestress Member Forces |
As an example of an exogenous load, a sign weighing 10 pounds (44 Newtons) is suspended from two corners of the prism, and . Along with the sign, a counterweight of 10 pounds (44 Newtons) is suspended from the tendon attachment point . This load and its effect on the prism are diagrammed in Figure 7.3. The tendons of the prism are linearly elastic, and a load of 20 pounds (89 Newtons) extends a tendon by 2%. The prism is supported at five of the six strut corners it rests on. One corner is excluded since it pulls away from the support surface by about 0.01 inch (250 μm) when the load is applied.

Figure 7.3: Orthogonal T-Prism: Positions and Effect of Exogenous Loads |
A two-sided numerical differentiation differential of 0.00001 (inches or pounds as appropriate) results in convergence in four iterations to a solution with a tolerance of 0.0001 (again inches or pounds as appropriate). Table 7.19 gives the displacements of the points where the exogenous loads are applied. Table 7.20 gives the reaction forces corresponding to the five corners where the prism is supported. Table 7.21 gives the forces and torques corresponding to each strut. Table 7.22 gives the forces and lengths corresponding to each tendon. And Table 7.23 gives the new coordinate values for the strut end points and the vector offsets to the tendon attachment points.
| ||||||||||
|
Table 7.19: Orthogonal T-Prism: Displacements due to Exogenous Loads |
| ||||||||||||||||||
|
Table 7.20: Orthogonal T-Prism: Support Reaction Forces due to Exogenous Loads |
| |||||||||||||||
|
Table 7.21: Orthogonal T-Prism: Strut Forces and Torques with Exogenous Loads |
| |||||||||||||||||||||||||||||||||
|
Table 7.22: Orthogonal T-Prism: Tendon Forces and Lengths with Exogenous Loads |
| ||||||||||||||||||||||||||||||||||||||||||||
|
Table 7.23: Orthogonal T-Prism: Coordinates with Exogenous Loads | ||||||||||||||||||||||||||||||||||||||||||||
10 If the member is of uniform cross section along its length, then is Young's modulus of elasticity of the material composing the member multiplied by the cross-sectional area of the member. See Vilnay90, p. 27. For materials where Young's modulus is variable, a more complex equation, or lookup table, for determining as a function of deviation of the member length, , from the reference length, , may be necessary.
11 See, for example, Hibbeler98, pp. 193-194, for a statement of the conditions for rigid-body equilibrium.
12 See, for example, Hibbeler98, pp. 138-141.