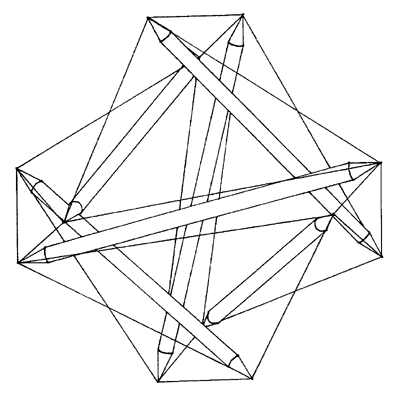
Figure 4.1: 2ν Diamond T-Tetrahedron
A Practical Guide to Tensegrity Design
Table of Contents
4.1 Higher-Frequency Spheres:
Introduction
4.2 Diamond Structures
4.2.1 Diamond Structures: Descriptive Geometry
 Figure 4.1: 2ν Diamond T-Tetrahedron |
As described in Section 2.3, diamond structures are characterized by the fact that each tendon triangle is connected to adjacent tendon triangles via one strut and two interconnecting tendons. This section examines a diamond configuration of the tensegrity tetrahedron. The zig-zag configuration of the 2ν1 t-tetrahedron was examined in Section 2.4. The diamond configuration of the 2ν t-tetrahedron is illustrated in Figure 4.1. It is topologically identical to the t-icosahedron (Figure 2.5 of Section 2.3). The only difference is that the tendon triangles of the 2ν diamond t-tetrahedron are two different sizes. The t-icosahedron is actually a special case of the 2ν diamond t-tetrahedron with all tendons the same length.
To review the contrast between the diamond and zig-zag configurations presented in Section 2.3, it is most productive to focus on the group of four small triangles from the 2ν diamond t-tetrahedron. These correspond to the 2ν zig-zag t-tetrahedron's four tendon triangles. If two tendon triangles from this group are considered to be facing each other nose-to-nose, the strut can be seen to connect the right ear of one triangle with the right ear of the other triangle as it did in the zig-zag t-tetrahedron. However, there are now two tendons interconnecting the two tendon triangles instead of just one. Each connects the right ear of one tendon triangle with the nose of the other.
These two tendons are symmetrical to each other, so the problem still consists of minimizing one length as it did in the original zig-zag problem, and even the same geometrical model as was used to solve that problem could be used here. However, the general case is more complex than this and is not amenable to treatment with models such as were used to examine the simple zig-zag t-tetrahedron. So to illustrate the general procedure, calculations are done for a frequency-four (or 4ν for short) diamond t-tetrahedron.
 Figure 4.2: 4ν Breakdown of Tetrahedron Face Triangle |
 Figure 4.3: 4ν Tetrahedron Face Triangle Projected on to a Sphere |
It is called a 4ν structure because its geometry derives from the 4ν geodesic subdivision of the tetrahedron.2 Only even-frequency subdivisions are used in tensegrity designs. Figure 4.2 shows a 4ν breakdown of a triangle, in this case the face of a tetrahedron. The labels and indicate which triangles are symmetrically equivalent. The heavy lines represent the lines of the geodesic breakdown used in the tensegrity design. Kenner's procedure is followed and these triangles are projected onto a sphere circumscribing the tetrahedron (see Figure 4.3). Notice that, considering symmetry transformations, there are two types of tendon triangles composing the system, an equilateral tendon triangle and an isosceles one.
 Figure 4.4: 4ν Diamond T-Tetrahedron: Representative Struts |
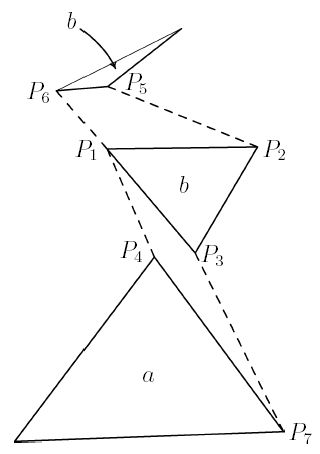 Figure 4.5: 4ν Diamond T-Tetrahedron: Representative Tendons |
Next the interconnecting struts and tendons are introduced. Figure 4.4 shows representative examples of the interconnecting struts. There are two types of strut. One type connects adjacent isosceles triangles, the other type connects isosceles with equilateral triangles. Figure 4.5 shows the corresponding interconnecting tendons. There are a pair of tendons corresponding to each strut type. Note that in both the figures, the triangles are skewed toward their final positions for clarity's sake. In the tensegrity programming problem, the sum of second powers of the lengths of the four diamond tendons are minimized, while the lengths of the struts and triangle tendons are considered constraints.
4.2.2 Diamond Structures: Mathematical Model
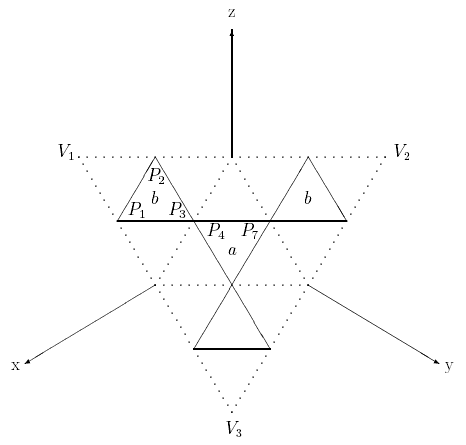 Figure 4.6: 4ν Diamond T-Tetrahedron: Coordinate Model (Face View) |
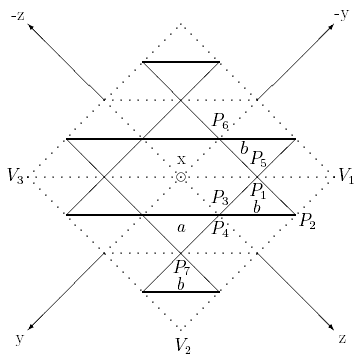 Figure 4.7: 4ν Diamond T-Tetrahedron: Coordinate Model (Edge View) |
Figures 4.6 and 4.7 show a tetrahedron inscribed within Cartesian coordinate space in a convenient orientation. With this orientation, any symmetry transformation of the tetrahedron can be accomplished merely by permuting the coordinate axes. On the tetrahedral face which falls in the positive quadrant (but extends into three others as well), the elements of the 4ν geodesic subdivisioning relevant to tensegrities have been inscribed. On this triangle, there are four points labeled , , and . , and represent the vertices of the isosceles triangle (or at least it will be isosceles when these points are projected onto a sphere); is a point on the equilateral triangle.
With these four points, all of the other points of the 4ν subdivisioning can be generated by using the symmetry transforms of the tetrahedron. Notice that, although geodesic structures exhibit mirror symmetry frequently, tensegrity structures generally do not. So cannot be generated from using a mirroring operation. Also, initially and coincide since initially the vertices of the isosceles and the equilateral triangle are in contact. When the computations start though, they part company.
The four points, , , and , can be generated from the three vertex points, , and , of the triangular tetrahedron face as follows:
|
|
Thus, the coordinates of , and summarized in Table 4.1 imply the coordinate values of , , and summarized in Table 4.2.
| ||||||||||||||||||||
|
Table 4.1: Tetrahedron Face: Vertex Coordinate Values | ||||||||||||||||||||
| ||||||||||||||||||||||||
|
Table 4.2: 4ν Diamond T-Tetrahedron: Unprojected Point Coordinates | ||||||||||||||||||||||||
When the values for , , and are projected onto the unit sphere, Table 4.3 is obtained. These coordinates serve as the initial values for the computation process. From them, the initial values of all member lengths are computed.
| ||||||||||||||||||||||||
|
Table 4.3: 4ν Diamond T-Tetrahedron: Projected Point Coordinates | ||||||||||||||||||||||||
In order to express all the members of the tensegrity, three more points are needed, , and . These points are symmetry transforms of , and respectively. and are obtained from and by a left-hand rotation of the tetrahedron about the vector from the origin to . In this coordinate system, this is achieved by taking the axis into the axis, the axis into the axis, and the axis into the axis, so that and can be expressed respectively as and .3 is obtained from by a left-hand rotation of the tetrahedron about the vector from the origin to the point . This is achieved by taking the axis into the axis, the axis into the axis, and the axis into the axis, so that can be expressed as .
So whenever coordinates for , or are required, these transformed versions of , or are used. Thus the symmetry constraints of the programming problem are implicitly subsumed in these expressions for , and . The variables of the programming problem are still limited to the coordinates of the original four points, and no new constraints need to be added to take into account symmetry.
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
Table 4.4: 4ν Diamond T-Tetrahedron: Initial Member Lengths |
Table 4.4 summarizes the initial lengths for the constrained members obtained using these coordinate values. The relevant mathematical programming problem is:
This completely specifies the problem. Again, only the coordinates of , , and are variables in the minimization process since the coordinates of , and are specified to be symmetry transforms of the coordinates of these points.
This is a very formal statement of the problem, and, as stated in Section 3.2, to solve it the inequality constraints are assumed to be met with equality.
4.2.3 Diamond Structures: Solution
As described in Section 3.2, the partials of the member equations and the non-member constraint equations can be conceived as a matrix, , which has as many rows as their are equations (10 in this case) and as many columns as there are coordinate values (12 in this case). The element of this matrix, , is the derivative of the equation with respect to the coordinate value. The coordinate values are numbered in the order they appear, so for example, is the partial derivative of the second power of the length of the tendon with respect to . Its value is . This partial is unusual in that it has two terms. Most of the member-equation partials are either zero or consist of a single difference.
The first step is to reformulate this as an unconstrained minimization problem by choosing a subset of the coordinates to be dependent coordinates whose values are obtained by solving the constraints given the values for the independently specified coordinates. Since there are six constraints, there are six dependent coordinates. This leaves six independent coordinates. By coincidence, the number of independent coordinates is equal to the number of dependent coordinates in this problem. Using Gaussian elimination with double pivoting on the partial derivative matrix for the system resulted in , , , , and being used as the initial independent coordinates. So, given the values for these coordinates, the constraints were solved for the remaining dependent coordinates, , , , , and .
| ||||||||||||||
|
Table 4.5: 4ν Diamond T-Tetrahedron: Initial Objective Function Derivatives |
The initial derivatives of the objective function with respect to the independent coordinates are summarized in Table 4.5. At a minimum point, the values of all these derivatives will be as close to zero as the accuracy of the computations permits. Instead of constantly looking at this whole list of derivatives (which can be very long for a complex structure) to assess how close to a minimum the system is, two summary statistics can be examined, the geometric average of the absolute values of these derivatives, and the variance of the natural logarithm of (the absolute value of) these derivatives. The variance is an important statistic, since if the system starts going singular, one or more of the derivatives starts to diverge from the rest. This singularity is a signal that the partitioning of variables between independent and dependent variables needs to be redone.
The value of the objective function is initially 1.86923. The system is solved using the Parallel Tangents technique which results in an objective function value of 1.65453. Table 4.6 summarizes the corresponding point values, and Table 4.7 summarizes the lengths of the members in the objective function thus obtained.
| ||||||||||||||||||||||||
|
Table 4.6: 4ν Diamond T-Tetrahedron: Preliminary Coordinate Values | ||||||||||||||||||||||||
| ||||||||||
|
Table 4.7: 4ν Diamond T-Tetrahedron: Preliminary Objective Member Lengths |
This would be the end of the calculations, except that when the endogenous member forces are calculated, they indicate that "tendon" is marginally in compression (see Table 7.1). This problem stems from the substitution of equalities for inequalities in the constraints. If inequalities had been used, this particular constraint would be found to be not effective. At this point the problem is dealt with by eliminating the member from the constraints which means the tendon doesn't appear in the final structure.4 Eliminating this constraint also means a new selection of independent variables needs to be made since seven are now needed. Repartitioning results in being added to the independent variables. Using the Parallel Tangents technique on this problem resulted in a final objective-function value of 1.65174. Table 4.8 summarizes the corresponding point values; Table 4.9 summarizes the objective function member lengths, and Figure 4.8 shows the final design where the location of the omitted tendon is indicated by a dashed line.
| ||||||||||||||||||||||||
|
Table 4.8: 4ν Diamond T-Tetrahedron: Final Coordinate Values | ||||||||||||||||||||||||
| ||||||||||
|
Table 4.9: 4ν Diamond T-Tetrahedron: Final Objective Member Lengths |
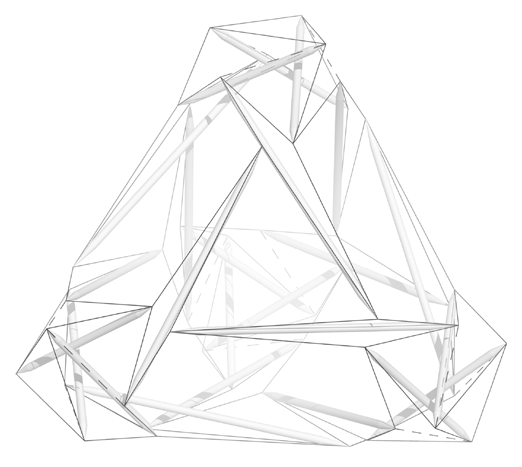 Figure 4.8: 4ν Diamond T-Tetrahedron: Final Design |