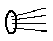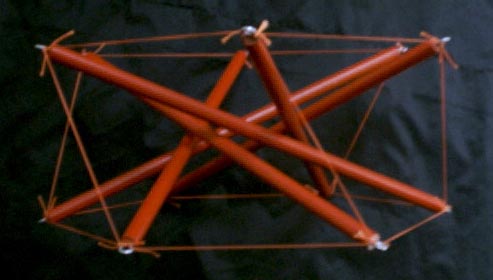
(VRML Model)

In June, 1999, I got into an e-mail discussion with Roger Tobie about equi-tendoned tensegrity prisms. This idea was originally introduced to me by Hugh Kenner in his book Geodesic Math. Kenner developed the idea in his thorough discussion of tensegrity prisms where he "proved" that tensegrity prisms with all tendon lengths equal were only possible for three-fold, four-fold and five-fold prisms.
Roger, whose 1967 Princeton thesis Kenner quotes for other purposes, contested this claim when I told him about it saying that he had built greater-than-six-fold equi-tendoned prisms successfully. I checked through Kenner's math again, and it all checked out, but Roger was insistent. He finally sent me a copy of his thesis which had illustrations of the models he'd built, none of which contradicted Kenner's claim since their side tendons were longer than the end tendons.
Roger was insistent enough though that before the thesis arrived I thought a little harder and realized one could make prisms where the side tendons skipped a strut instead of connecting adjacent struts. This changed the math and allowed a six-fold equi-tendoned prism to be built. Higher-fold equi-tendoned prisms are possible as well. At higher and higher symmetries, more struts have to be skipped by the side tendons and the struts must be thinner and thinner to avoid running into each other.
I only got around to building a model in May, 2003. It is interesting to contemplate. I'll include a couple more views below, but there are many more angles of interest. It's also available on the tensegrity viewer as "6-Fold Equi-tendoned Prism".
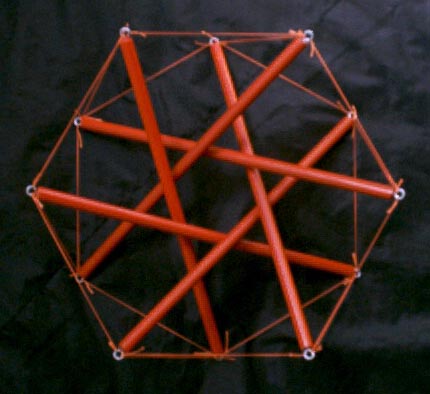
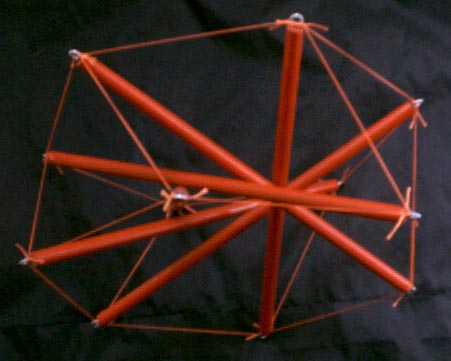
Earlier in 2003, I finally got around to reading Roger's thesis. Its hands-on operational logic is a marvel to behold, and it has a well-annotated bibliography. Hopefully it will get on-line at some point. In 2004, I found David Georges Emmerich presented this structure in his 1988 book Structures Tendues et Autotendantes (see his "antiprisme hexagonal" on p. 84).