 |
 |
 |
(VRML Model)
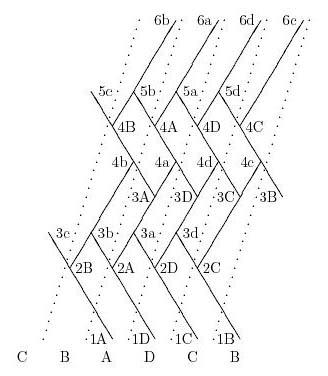
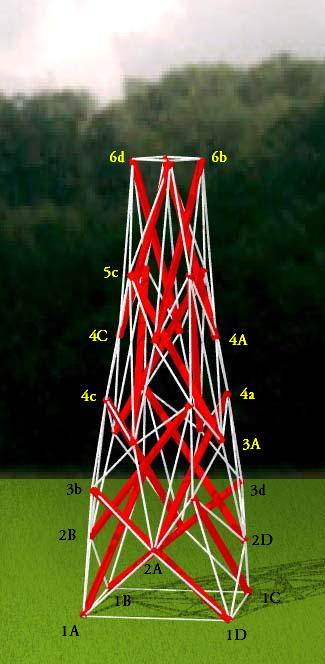
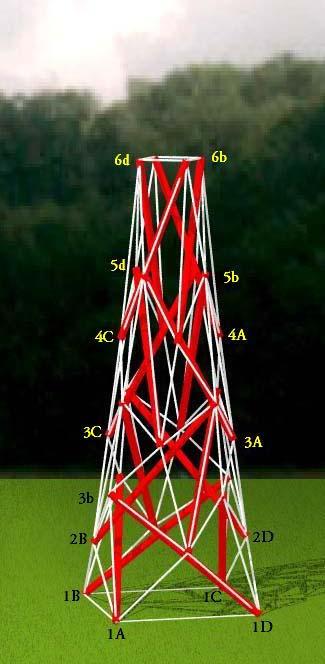
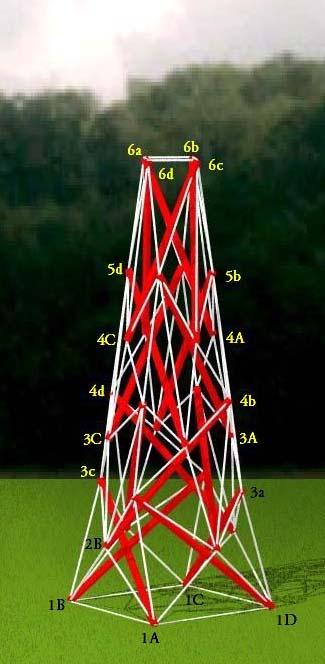
Schematic for the Triangulated Tensegrity Obelisk
Member Descriptions
[name, end point names, weight (if in objective function),
second power of length (if a constraint), member category,
Obj/Con/Exc (put in objective function, use as a constraint or
exclude from computations), flags]
For assembly purposes, only the name and end point names are
of interest. The other information may be of interest after
A Practical Guide to Tensegrity Design has been consulted.
<Member> strut13 pt1A pt3c 0.0 sqr(2.5) 1 Con CalcClear Inelastic *
<Member> strut24 pt2A pt4a 0.0 sqr(2.5) 1 Con CalcClear Inelastic *
<Member> strut35 pt3A pt5c 0.0 sqr(2.5) 1 Con CalcClear Inelastic *
<Member> strut46 pt4A pt6a 0.0 sqr(2.5) 1 Con CalcClear Inelastic *
<Member> baset pt1A pt1B 0.0 sqr(1.9) 2 Con *
<Member> apext pt6a pt6b 0.0 sqr(1.9/3) 2 Con *
<Member> guy1 pt1A pt3b 0.10 0.0 3 Obj *
<Member> guy2 pt2A pt4b 0.20 0.0 3 Obj *
<Member> guy3 pt3A pt5b 0.20 0.0 3 Obj *
<Member> guy4 pt4A pt6b 0.10 0.0 3 Obj *
<Member> TL12 pt1A pt2B 0.9 0.0 4 Obj *
<Member> TS23 pt2A pt3b 1.0 0.0 4 Obj *
<Member> TL23 pt2A pt3A 0.9 0.0 4 Exc *
<Member> tS23 pt2A pt3a 1.0 0.0 4 Obj *
<Member> TS34 pt3A pt4a 1.1 0.0 4 Obj *
<Member> TL34 pt3A pt4B 0.9 0.0 4 Exc *
<Member> tS34 pt3A pt4b 1.1 0.0 4 Obj *
<Member> tL34 pt3a pt4a 0.9 0.0 4 Exc *
<Member> TS45 pt4A pt5b 1.2 0.0 4 Obj *
<Member> tS45 pt4A pt5a 1.2 0.0 4 Obj *
<Member> tL45 pt4d pt5a 0.9 0.0 4 Exc *
<Member> tL56 pt5a pt6a 0.9 0.0 4 Obj *
<Member> tT33 pt3a pt3A 2/3 0.0 4 Obj *
<Member> tT44 pt4a pt4A 2/3 0.0 4 Obj *
<Member> xtr1 pt1A pt2A 0.1 0.0 7 Obj *
<Member> xtr2 pt2A pt3B 0.1 0.0 7 Obj *
<Member> xtr3 pt3A pt4A 0.1 0.0 7 Obj *
<Member> xtr4 pt3a pt4b 0.14 0.0 7 Obj *
<Member> xtr5 pt4a pt5a 0.10 0.0 7 Obj *
<Member> xtr6 pt5a pt6b 0.12 0.0 7 Obj *
In-Situ Member Lengths
These are the lengths of the members when they are in place
and prestress is applied. These are raw unadjusted values and
assume the hubs of the structure are single points. The
values are in model units.
strut13: 2.5 strut24: 2.5 strut35: 2.5
strut46: 2.5 baset: 1.9 apext: 0.633333
guy1: 1.66175 guy2: 1.96864 guy3: 2.23353
guy4: 2.3468 TL12: 1.30665 TS23: 1.27839
TL23: 1.70955 tS23: 0.878816 TS34: 0.919302
TL34: 1.5853 tS34: 0.776239 tL34: 1.79612
TS45: 1.00054 tS45: 0.997978 tL45: 1.88638
tL56: 1.55026 tT33: 0.91207 tT44: 0.901221
xtr1: 1.26893 xtr2: 1.40126 xtr3: 1.34063
xtr4: 1.19009 xtr5: 1.66707 xtr6: 1.47086
Relative Member Prestress Force Magnitudes
These values are useful for developing an assembly
strategy for the structure. The tighter tendons are much
easier to tie in place early on, while the looser tendons
can be left to the last. This information is also used
to adjust tendon lengths since the measured length of a tendon
will be shorter for a highly-stressed tendon with the same
in-situ length as a tendon which is not so stressed.
strut13: -1.61292 strut24: -1.43981 strut35: -1.41234
strut46: -1.83596 baset: 0.286017 apext: 0.229667
guy1: 0.166175 guy2: 0.393727 guy3: 0.446705
guy4: 0.23468 TL12: 1.17599 TS23: 1.27839
tS23: 0.878816 TS34: 1.01123 tS34: 0.853863
TS45: 1.20065 tS45: 1.19757 tL56: 1.39523
tT33: 0.608047 tT44: 0.600814 xtr1: 0.126893
xtr2: 0.140126 xtr3: 0.134063 xtr4: 0.166612
xtr5: 0.166707 xtr6: 0.176504
Average tendon force magnitude: 0.584931
Construction Lengths (in inches, 16ths and 32nds)
The construction length of a tendon is less than the in-situ
length since when the tendon is measured off it isn't under
any prestress force. The construction length for a member
represents the distance between the locations where it
departs from the hub. The struts were cut from
1-inch by 1-inch hardwood garden stakes. The tendon material
was single strands of twisted #18 nylon twine. Its behavior
under stress is highly non-linear, so a look-up table
was used to compute strains. Prestress forces were assumed
to affect tendon lengths and not strut lengths.
Average Tendon Force Magnitude (chart units)> 20
Length Scale Factor> 48/2.5
Strut and Tendon Hub Adjustments> 0 0.5
(adjust the tendon lengths by subtracting a half inch from
both ends)
strut13: 48 0 0 strut24: 48 0 0 strut35: 48 0 0 strut46: 48 0 0
baset: 34 6 0 apext: 10 14 0 guy1: 30 5 0 guy2: 35 5 1
guy3: 40 1 0 guy4: 42 13 1 TL12: 22 0 1 TS23: 21 7 0
tS23: 14 12 0 TS34: 15 6 0 tS34: 12 15 0 TS45: 16 10 0
tS45: 16 9 1 tL56: 26 1 0 tT33: 15 9 1 tT44: 15 6 1
xtr1: 23 0 0 xtr2: 25 7 1 xtr3: 24 5 1 xtr4: 21 7 0
xtr5: 30 6 1 xtr6: 26 11 0
Material Quantities
This provides an estimate of how much material will
be needed to assemble the structure, in this case
inches of garden stake and inches of nylon twine.
The lengths must be adjusted to take into account the
fact that the strut extends past the hub and some length
of tendon is required to tie it to the strut.
The Quantity value given for each Type does not take
into account the Cross Section value. The total
Quantity given across from Tndns does.
The Cross Section value of 2 indicates all the twine
strands were doubled up, i.e. two lengths of twine were
used to tie each tendon.
Length Scale Factor> 48/2.5
Strut and Tendon Adjustments> (-1) (-6)
Cross
Type Section Quantity Count
1 1 800 16
2 2 281.872 8
3 2 795.605 16
4 2 1187.24 40
7 2 911.798 24
Strts 800 16
Tndns 6353.02 88
 |
 |
 |

Schematic for the Triangulated Tensegrity Obelisk
structure file: obelisk3/x4l4obelisk1.rc
variable file: obelisk3/x4l4obelisk1.dat
stress-strain chart file: v04oct_d/wellingtn.ssc
digit list file: src/standard.dls
cross-section table: src/double.cst
|
CONTACT:
Bob Burkhardt
Tensegrity Solutions
Box 426164
Cambridge, MA 02142-0021
USA
e-mail: bobwb@juno.com