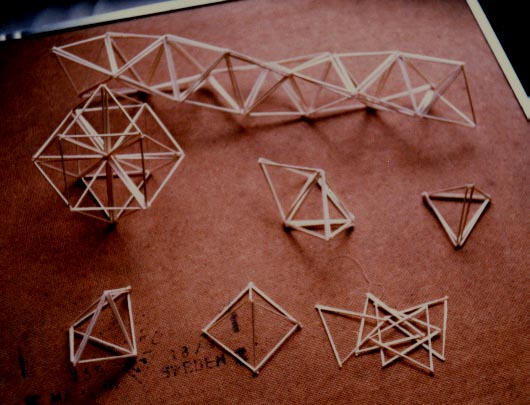
Here are some interesting things which can be built with toothpick triangles. By far the most interesting is the tetrahelix at the top. It is fascinating to watch it form as it is built up. It is one of the first models I remember building. This was back in my graduate student days. A display of Bucky's Tetrascroll outside MIT's Hayden Library was what prompted me to construct it. It was in a case and was unfolded week by week until all the pages were displayed. I had to build a model to understand what he was drawing, or at least to verify the puzzling claims he was making about what could be constructed by pasting tetrahedra together.

Bucky originally made Tetrascroll in 1975 as a lithographic creation at Universal Limited Art Editions. Later (1982) it came out as a book published by ULAE, Inc., and St. Martin's Press, and I quickly got a copy.

A close runnerup for interest is the vector equilibrium (middle row to your far left). It is sometimes confused with the cuboctahedron, but the internal structure is important here. Its external edges do indeed outline the edges of a cuboctahedron. All its edges have the same length as its radius. Two-Frequency Vector Equilibrium is a photo of a model of the 2ν version.

Even building a tetrahedron was highly instructive. I was accustomed to think of it as a sort of pyramid, but it has a variety of other interesting axes, like the one that goes through the midpoint of two opposite edges. I first appreciated this with a model in hand, but perhaps exploring it on the Tensegrity Viewer (it's not a tensegrity, but I put in on the list of structures anyway) will also point this out.

Anything which can be built from toothpicks can be built from equal-diameter spheres as well. Each corner of a triangle now becomes the center of a sphere, and the diameter of the spheres used becomes the determiner of scale rather than the length of the toothpicks. Sphere Models, the next photo in this gallery, shows how things look when spheres are used to construct models like these.