On August 14, 1982, I bought a bicycle tire rim at a yard sale in Danvers, Massachusetts, so I could test Bucky's proposition that it takes a minimum of twelve spokes to secure the hub in relation to the rim (see Fig. 640.41A from Synergetics entitled "Stabilization of Tension: Minimum of 12 Spokes"). The rim originally had 36 spokes.
The next day I took out all the spokes and went through, starting with one spoke, and looked to see what the smallest number of spokes was which would firmly secure the orientation of the hub with respect to the rim. I found eight spokes to be sufficient. In the pattern I assembled them in, they all seemed to be necessary. If I removed one, the hub could be reoriented with respect to the rim.
Below is a closeup view of the hub. It is attached to the rim in a pattern strikingly similar to the way a diamond tensegrity strut is attached to its mother structure. (A diamond tensegrity strut is also attached with eight tendons.)
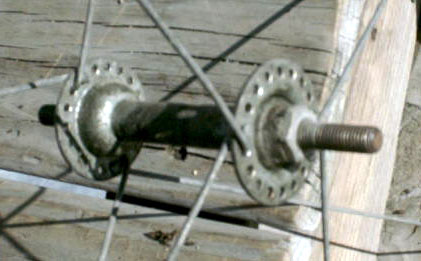
The rim made a great gyroscope and was useful in my later studies of precession. Later (April 17, 2003), I repositioned the spokes so they were distributed more evenly. A photo of the new arrangement:

Below is a closeup view of the rearranged hub. I found I needed to introduce a one-hole gap at two locations to make this new arrangement work. Later I found I could assemble things without the gap.

Doug Milliken, who participates in the Geodesic listserv, for awhile advocated a six-spoke wheel with three spokes spiraling one way on one side of the hub and three spiraling the other way on the other side of the hub. He built a model, and I tried it out on my wheel. We both agreed there were degrees of freedom which disqualified this approach though both of our models had defects in construction which made them less than ideal as test vehicles. Doug also notes that the definitive book on the bicycle wheel is The Bicycle Wheel (3rd edition) by Jobst Brandt (Avocet, Inc., Palo Alto, California).
Another resource which randome inventor Dick Fischbeck, who also participates in the Geodesic listserv, pointed out are the pages where Amy Edmondson addresses this issue in A Fuller Explanation. Amy starts with a six-spoke wheel and builds it up to a twelve-spoke wheel.
My theory about how I got away with eight spokes when other people have required twelve rests on the fact that the spokes cross in a pairwise fashion. This is an additional constraint because it inhibits rotation of the hub in one direction since the spokes can't go through each other. The opposite pair of spokes on the same side of the hub cross in a way that inhibits rotation in the other direction. The axis of rotation is the line connecting the two locations on the one side of the hub where its four spokes are attached. The other side of the hub adds another two constraints to resist rotation about yet another axis. Four pairs of spokes make four additional constraints which make twelve altogether.
It would have been easy to assemble the eight-spoker so I only got ten constraints and then I'd have probably found the hub wasn't so firmly set and avoided this whole controversy. It is interesting that the constraint imposed by two crossing spokes has much the same character as that imposed by a single spoke. It is an inequality that allows rotation in one direction but not the other. A spoke allows movement which bends the spoke but not movement which would extend the length of the spoke. Both constraints can be mathematically expressed as inequalities. This shows Fuller's wisdom in choosing his granularity for constraining a system at twelve. Many times I hear talk of six degrees of freedom which need to be constrained. In these cases, constraints are expressed as equalities. In some of these cases I imagine some more effective constraint possibilities get overlooked because the employment of inequality constraints is not explored. And why not mention synergy as well? Here is a situation where 1 + 1 = 3. Two spokes, which individually amount to a single constraint each, in combination impose three constraints.
On October 16, 2006, I reassembled the eight-spoker and then reversed two sets of two spokes so the crossings duplicated existing constraints rather than introducing new ones. This is the ten-constraint arrangement discussed in the previous paragraph. I didn't notice any difference in firmness between this arrangement and the twelve-constraint arrangement. This result casts a doubt in my mind on my explanation in the paragraph before last and increases my interest in trying the seven-spoke arrangement Tim Tyler recently introduced on his page. Note that some of the spokes in his seven-spoke arrangement have a shorter rim-to-hub distance than spokes in a stock configuration. He obtained this by reversing the spoke nipples on those spokes. When I tried it, that wasn't quite enough to give me short enough distances with my spoke/rim/hub collection.
This experience demonstrates to me the benefits of going into an experiment without preconceived notions about the outcome, or at least with the idea firmly in mind that one is testing a hypothesis which could turn out to be false even though it seems very reasonable based on past experience. It is also an example of the little twists of reality that frustrate armchair generalization. Building a model can be very instructive.
In addition to introducing a seven-spoke arrangement, Tim Tyler has confirmed my results. He takes things a step further than I would: he thinks only eight inequality constraints are necessary even in the "me"-ball experiment ( Fig. 401.00 from the "Drawings Section" of Synergetics). Instead, I think his ball-and-chair experiment points to how twelve tensors could be organized when an octahedron rather than a tetrahedron is used for support.
While removing one spoke in the eight-spoke arrangement introduces a palpable flexibility which isn't present in the eight-spoke arrangement, it is possible that there is a further eleven/twelve threshold beyond this seven/eight threshold which can only be detected by means of a stilson wrench of the sort Bucky used.